LeetCode 99 - Recover Binary Search Tree

發表於 分類於 Disqus：

題目

題目連結：https://leetcode.com/problems/recover-binary-search-tree/

給定一個二元搜尋樹，恰好有兩個點被交換了。

復原出原本的二元搜尋樹。

範例說明

Example 1:

1
2
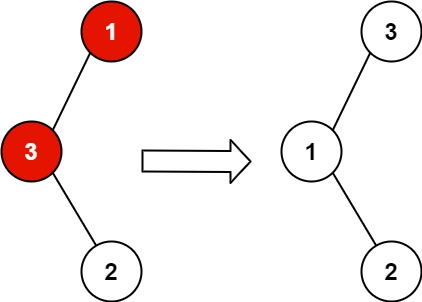
3
Input: root = [1,3,null,null,2]
Output: [3,1,null,null,2]
Explanation: 3 cannot be a left child of 1 because 3 > 1. Swapping 1 and 3 makes the BST valid.

Example 2:

1
2
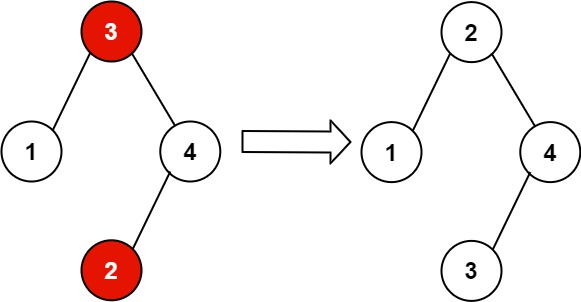
3
Input: root = [3,1,4,null,null,2]
Output: [2,1,4,null,null,3]
Explanation: 2 cannot be in the right subtree of 3 because 2 < 3. Swapping 2 and 3 makes the BST valid.

想法

二元搜尋樹的其中一個重要的性質就是其中序走訪（Inorder Traversal）恰好就是排序好的序列。

知道這個性質後，對這個錯誤的搜尋樹使用中序走訪，可以得到一個序列，因為兩個點恰好被交換，因此得到的序列中應該是排序好的，但是恰好有兩個數字被交換了。

題目因此可以變成『給一個排序好的序列，裡面恰好有兩個數字被交換，找出原本的序列』。

考慮一個 1 ~ 9 排序好的序列，有兩種交換數字的可能性：

  1. 交換的數字是相鄰的
    Example：交換 3, 4。

    1
    2
    1 2 3 4 5 6 7 8 9
    1 2 4 3 5 6 7 8 9

    可以發現，相鄰兩數交換後會產生一個相鄰的逆序數對{(4, 3)}，復原這個逆序數對即可。

  2. 交換的數字是不相鄰的
    Example: 交換 3, 7。

    1
    2
    1 2 3 4 5 6 7 8 9
    1 2 7 4 5 6 3 8 9

    可以發現，不相鄰兩數交換後會產生兩個相鄰的逆序數對 {(7, 4), (6, 3)}，則交換第一個逆序數對中的前面的數，以及第二組逆序數對中後面的數即可。

統整來說，我們可以在中序走訪中尋找相鄰的逆序數對，如果有一組的話，則交換逆序數對的兩數；如果有兩組的話，則交換第一組逆序數對前面的數以及第二組逆序數對後面的數。

實作細節

利用 lastNode 紀錄上一個點，若 lastNode == nullptr 代表當前是根節點，否則當 lastNode->val > root->val 時，則逆序數對產生。

利用 target 紀錄第一組逆序數對中的第一個數字，利用 candidate 紀錄要與 target 交換的另一個數。

因此，在遇到第一組逆序數對時，先假設只有一組，也就是 candidate 應該等於第一組逆序數對的第二個數字，也就是當前的節點 root 的數字，並繼續走訪。當遇到第二組逆序數對時，可以知道 candidate 應該要換成第二組逆序數對後面的數，也就是當前的節點 root。

最後遍歷完成後交換 target, candidate 上的值即可。

程式碼

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
/**
 * Author: justin0u0<[email protected]>
 * Problem: https://leetcode.com/problems/recover-binary-search-tree/
 * Runtime: 32ms
 */

/**
 * Definition for a binary tree node.
 * struct TreeNode {
 *     int val;
 *     TreeNode *left;
 *     TreeNode *right;
 *     TreeNode() : val(0), left(nullptr), right(nullptr) {}
 *     TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
 *     TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}
 * };
 */
class Solution {
public:
  TreeNode* lastNode = nullptr;
  TreeNode* target = nullptr;
  TreeNode* candidate = nullptr;

  void solver(TreeNode* root) {
    if (root == nullptr)
      return;

    solver(root->left);

    if (target == nullptr && (lastNode != nullptr && lastNode->val > root->val)) {
      // target == nullptr，第一組相鄰逆序數對
      target = lastNode;
      candidate = root;
    } else if (target != nullptr && (lastNode != nullptr && lastNode->val > root->val)) {
      // target != nullptr，第二組相鄰逆序數對
      candidate = root;
    }
    lastNode = root;

    solver(root->right);
  }

  void recoverTree(TreeNode* root) {
    solver(root);
    // 遍歷完成，交換 `target`, `candidate` 上的數值
    swap(target->val, candidate->val);
  }
};